Infinite Money Glitch: How MicroStrategy Convertible Bonds Profit from Gamma Trading — Part 3

Infinite Money Glitch: How MicroStrategy Convertible Bonds Profit from Gamma Trading — Part 3

The world of convertible bonds may sound like a convoluted financial labyrinth, but at its core lies a beautifully engineered strategy that turns market chaos into calculated profit. This is the “infinite money glitch” hedge funds leverage when dealing with MicroStrategy (MSTR) convertible bonds, fueled by the dynamic interplay of delta and gamma — two unassuming metrics that are the backbone of this lucrative game.

At its essence, delta measures how much a bond mimics stock price movements, while gamma shows how quickly this mimicry evolves. Together, they act as the hedge fund’s compass, enabling a constant recalibration of positions to capitalize on every price swing. Picture a Formula 1 driver — delta is the steering wheel, keeping the strategy on course, while gamma is the accelerator, propelling profits during market volatility.

Take MSTR, a poster child of volatility, and imagine a hedge fund snapping up its convertible bonds. Whether MSTR’s stock soars to $1,000 or plunges to $370, this strategy thrives by dynamically hedging short positions, profiting from the inefficiencies and price movements along the way. The mechanics are intricate, but the goal is simple: profit not from where the stock goes but how it gets there.

In this piece, we’ll unpack how hedge funds exploit these forces, using MSTR as a case study to reveal the alchemy of turning turbulence into triumph.

Understanding Delta and Gamma in Convertible Arbitrage: A Simplified Guide

Delta and gamma are the heartbeats of convertible arbitrage, dictating how hedge funds maneuver their positions to extract profits. They aren’t just abstract financial terms — they’re the tools that transform volatility into opportunity. Let’s break down these concepts into manageable pieces, so even those new to finance can appreciate the elegance of this strategy.

What is Delta?

Delta measures how much the price of a convertible bond changes when the stock price moves by $1. In simple terms, it tells you how much the bond behaves like the stock. For example:

• Delta of 0.5: The bond acts like owning half a share of the stock.
• Delta of 1: The bond behaves almost exactly like the stock (deep in-the-money).
• Delta of 0: The bond behaves purely like debt (far out-of-the-money).

Delta evolves dynamically based on market conditions and the relationship between the stock price and the bond’s conversion price (the price at which the bond can be converted into shares).

Key Influencers of Delta

1. Stock Price (S):
   As the stock price rises and nears the conversion price, delta increases because the conversion option becomes more valuable.
2. Conversion Price (K):
   If the stock price is far below the conversion price, delta is lower, reflecting a bond that acts more like traditional debt.
3. Time to Maturity (T):
   More time until maturity gives the stock price more opportunity to rise, increasing delta.
4. Volatility (σ):
   High volatility increases delta because the stock has a greater chance of exceeding the conversion price.
5. Risk-Free Rate (r):
   A higher risk-free rate slightly increases delta by enhancing the value of potential future payoffs.

What is Gamma?

Gamma measures how much delta changes when the stock price moves by $1. If delta is the speedometer of a car, gamma is the accelerator — indicating how quickly the speed (delta) changes.

• High Gamma: Indicates delta changes rapidly with stock price movements, offering frequent opportunities for adjustments.
• Low Gamma: Reflects slower changes in delta, reducing opportunities for profitable trades.

Gamma is the driving force behind dynamic hedging, enabling hedge funds to profit from fluctuations in stock prices regardless of direction.

How Delta is Calculated

For convertible bonds, delta is derived from the embedded call option within the bond. The calculation uses the Black-Scholes model or similar frameworks:

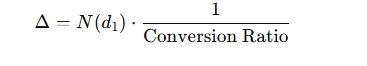
Where:

• N(d1)N(d_1)N(d1​): The delta of the embedded call option (from the Black-Scholes model).
• Conversion Ratio: Number of shares the bondholder can receive per bond, calculated as:

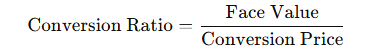
Black-Scholes Component

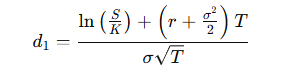
Here:

Example: Calculating Delta for MicroStrategy (MSTR) Convertible Bonds

Scenario:

• Stock Price (S): $800
• Conversion Price (K): $630
• Face Value: $1,000
• Volatility (σ): 50%
• Time to Maturity (T): 3 years
• Risk-Free Rate (r): 3%

Step 1: Calculate the Conversion Ratio

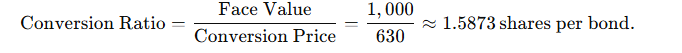
Step 2: Compute d1

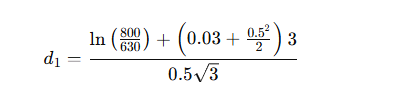
Step 3: Find N(d1)

From standard normal distribution tables:

• N(0.813)≈0.791

Step 4: Calculate Delta

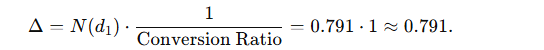
At $800, the bond has a delta of approximately 0.79, meaning it behaves like owning 79% of a share per bond.

Infinite Money Glitch: How MicroStrategy Convertible Bonds Profit from Gamma Trading — Part 3 was originally published in The Capital on Medium, where people are continuing the conversation by highlighting and responding to this story.